In a previous post, I broadly addressed the risk many individual investors ignore or do not understand while buying options as speculative investments – the impact of volatility on option values. This unique risk is mostly covered by the term vega, or the sensitivity of the option’s price to changes in volatility. Option traders have a love affair with greek letters of the alphabet when describing the risks embedded in options (though vega is not really a letter in the standard greek alphabet). The other most misunderstood “greek” is named gamma and is often the risk that trading desks diligently and tightly limit as a risk management practice.
Explanation of Vanilla Put and Call Options:
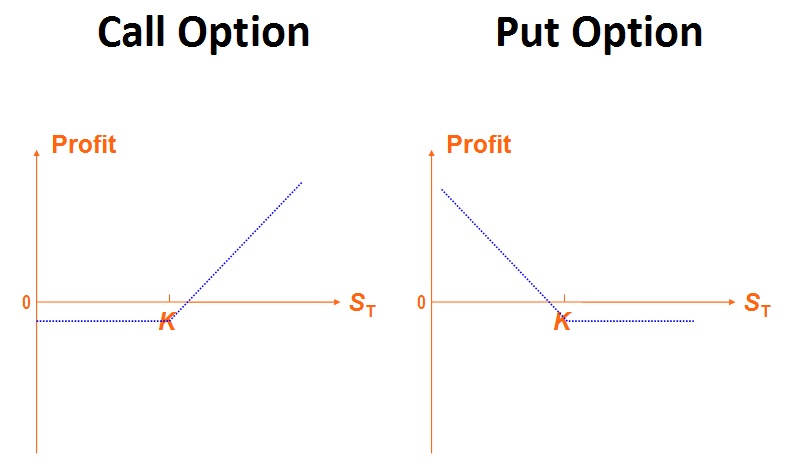
Let us first review the basics before we dive into the more complicated topic of what gamma means and how to limit it. Options are loved by many investors in part because they generally limit the amount of risk taken in a speculative bet. When buying a call option, the investor pays a fixed premium and receives profits when the stock goes above the predetermined strike price before the option’s expiration. With a put option, the investor pays a fixed premium and receives profits when the stock goes below the predetermined strike price before expiration. Quite simple and best shown in an illustration:
The analysis of the option’s value at expiration is rather simple as well. If you purchased a put option contract for 100 shares of IBM stock at a strike price of 90 and the stock price closes at 75 on expiration, then the payoff is (90-75)=$15 per share or $15*100=$1,500 in profit. The complexity of the option is apparent between the time you purchase/sell the option and the time that the option expires. Unlike buying/shorting a stock, an option’s contract has a non-linear payoff function. The non-linearity of the payoff function is represented by gamma, while the linear portion of the payoff is represented by delta.
For the remainder of this post we will express our problem with a simple example:
- Purchased put option on Yahoo (YHOO) stock (100 shares=1 contract) on October 21, 2009 for a premium of $3.20
- Strike of the put option = $17.50
- Expiration of the put option is January 21, 2011
- Current price of Yahoo is $17.20
- Yahoo does not currently pay a dividend
- Volatility will remain fixed at 40% and interest rates at 1%
The above graph demonstrates the curvature of the put option’s payoff and how it changes as time passes and as the stock moves along different prices. The maximum profit on this graph would be $8.50. To achieve this profit, the investor would need to sell the stock at the $17.50 strike price and have Yahoo’s price trade at $9 at expiration.
Explanation of Gamma:
The gamma represents the curvature of the option’s payoff curve. The below graph adds the curvature of the gamma over the option’s payoff curves. It demonstrates the put option’s upward spiking gamma as the option approaches its strike price and as its time to expiration dwindles. As we move closer and closer to expiration, the curvature of the gamma reaches its maximum, peaking at the option’s strike price. The peak occurs when the strike price and the underlying stock price converge.
Delta is the change in the price of the option contract for a given change in the price of the underlying stock. When the delta is .5, that means that the option value changes $.50 for every $1 change in the stock. The gamma is the rate of change of delta. We can understand this spike by thinking of this yahoo option 1 minute before expiration of the contract. If the stock is currently sitting at $17.60 one minute before expiration when the strike for the put option is at $17.50, the outcome is binary. Either the option is going to expire worthless or it is going to be in the money and profitable for the option buyer. That means that when the stock is trading around $18 the value of the option will not move very much, but as the stock approaches $17.50 the option delta accelerates (gamma) quickly until it reaches 1 and is trading dollar for dollar with the underlying stock.
Gamma risk is mostly ignored by individuals who are buying options, but it must be watched closely by those who are selling options either as an income generation strategy or for those who act as market makers, even if they delta hedge the linear risk multiple times a day. Gamma can escalate quickly, so it is a risk that should be managed closely for anyone wanting to be a net option seller.
Most individual investors sell options and do not delta hedge their exposures. The most popular strategies for individual investors are selling call spreads, butterflies, iron condors, or calendar spreads. Most of these option selling strategies exist and are promoted by trainers for the sole purpose of mitigating gamma losses. When selling a spread you strictly limit your losses to the cap of the upper option, but in doing so you are giving away a lot of the premium in the option that you are selling.
Gamma should only be scary for those who do not practice strong risk management. Stop giving away your profits by capping your losses and instead focus on developing your own risk management strategy. There are two key principles to stick to:
- Close out winning positions that have even a remote chance of ending in the money. If you sell an option for $1 per share, do not ride it through expiration for the last $.10 while risking massive losses if there is a dislocation in the market.
- Close out losing positions before they get out of hand. Holding on to losers and hoping for comebacks is a recipe for disaster. The gamma will continue to increase as you hold onto the losing trade and your risk profile continues to get uglier.